2009
11.02

Exponential functions

Category: Uncategorized / Tag: ,  / Add Comment

The function \emph{f} defined by f(x) = b^{x} where b, and the exponent x is any real number, is called an exponential function with base b

When you work with exponential functions, it may be necessary to apply rules for exponents. These rules are as follows, where m and n are real numbers and a and b are positive.

  • a^{m} \cdot a^{n} = a^{m+n}
  • \frac{a^{m}}{a^{n}} = a^{m-n}
  • (a^{m})^{n} = a^{m \cdot n}
  • (ab)^{n} = a^{n} b^{n}
  • (\frac{a}{b})^{n} = \frac{a^{n}}{b^{n}}
  • (a)^{1} = a
  • a^{0} = 1
  • a^{-n} = \frac{1}{a^{n}}

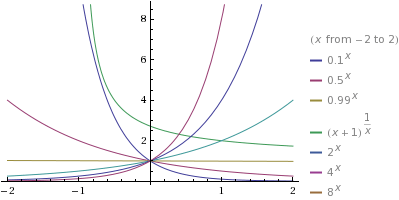
Graphs of some exponential functions are shown below:

plot .1^x, .5^x, .99^x, (1 + x)^(1/x), 2^x, 4^x, 8^x

plot .1^x, .5^x, .99^x, (1 + x)^(1/x), 2^x, 4^x, 8^x

Here, some properties of exponential functions

  • The domain of an exponential function is all real numbers
  • The range is all positive real numbers
  • Since b^{0} = 1 for every base b, each graph has y-intercept (0, 1). There is no x-intercept.
  • If b > 0, then y = b^{x} is an increasing function; its graph rises from left to right. Also

\mathop {\lim }\limits_{ x \to \infty } b^{x} = \infty and \mathop {\lim }\limits_{ x \to -\infty } b^{x} = 0


  • Thus as x \rightarrow -\infty, the graph has the x-axis as a horizontal asymptote.
  • If 0 < b < 1, then y = b^{x} is a decreasing function; its graph falls from left to right. Also

\mathop {\lim }\limits_{ x \to \infty } b^{x} = 0 and \mathop {\lim }\limits_{ x \to -\infty } b^{x} = \infty


  • Thus as x \rightarrow \infty, the graph has the x-axis as the horizontal asymptote.

No Comment.

Add Your Comment

Your Comment